Maths - Modelling with Differentiation
What would the differential be called for $A = \pi r^2$?
#####
\[A = \pi r^2 \\ \frac{dA}{dr}\]How would you describe the differential?? The rate of change of area with respect to radius.
\[A = \pi r^2 \\ \frac{dA}{dr}\]
How would you describe the differential?
The rate of change of area with respect to radius.
#####
\[V = \frac{4}{3} \pi r^3 \\ \frac{dV}{dr} = 4\pi r^2\]How could you explain “the rate of change of volume with respect to radius”?? How much additional volume you gain for a small change in the radius.
2021-01-29
Can you differentiate $V = \frac{4}{3} \pi r^3$?
\[V = \frac{4}{3} \pi r^3 \\ \frac{dV}{dr} = 4\pi r^2\]
How could you explain “the rate of change of volume with respect to radius”?
How much additional volume you gain for a small change in the radius.
#####
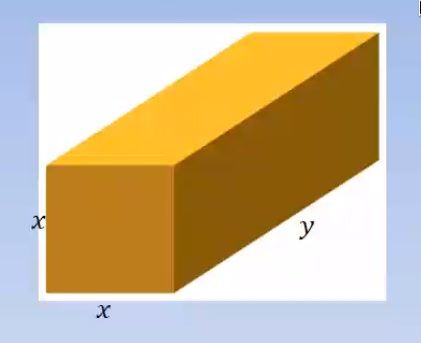
You have the two equations
\[A = 2x^2 + 3xy = 54m^2 \\ V = x^2y\]How would you find the actual volume of the cubiod?? Rearrange the first formula in terms of $y$ and then substitue back into the volume formula.